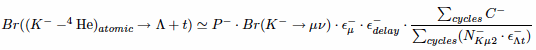Today's Progress 11. May 2010

2010 May 11th report - The evaluation of per-stopped-K^- formation rate of (⁴He-K^-)_atomic -> &Lambda + t four-nucleon absorption process (1)

As was discussed in the 2010 May 6th report, we have clearly observed the four-nucleon absorption process,

(⁴He-K^-)_atomic -> Λ + t (711.7 MeV/c).

Here, we try to evaluate the percent-per-stopped K^- branching fraction of the reaction. At the same time, we will perform a Monte-Carlo simulation of a similar reaction,

(⁴He-K^-)_atomic -> Σ⁰ + t (620.5 MeV/c),

to study proton momentum distribution as a possible physical BG of ⁴_ΛHe->t + p reaction if backward triton is required, assuming a similar branch to the Λt channel. The two-body momentum of triton/Y⁰ is calculated according to the particle mass tabulatted below.

Particle/Nucleus	mass (MeV/c²)
⁴He	3727.40841
K^-	493.677
t	2808.94327
&Lambda	1115.683
&Sigma⁰	1192.642

Normalization of the process and acceptance study

Now, we are interested in to estimate exactly the acceptance of a cascade reaction of a branch of four-nucleon absorption process,

(⁴He-K^-)_atomic -> Λ+t (711.7 MeV/c) ,

and successive weak decay of Λ,

Λ -> p + π^-.

The exact normalization is performed by

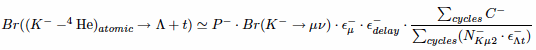
, where

P^- : Free-decay rate of atomic K^- by meta-stable states, 3.5 +- 0.5 %.

Br(K^- -> μ&nu) : Branching ratio to Kμ2 decay, 63.55 %.

&epsilon^-_&mu : Physical acceptance of &mu^-, 7.55 %.

&epsilon^-_delay : Muon survivability by delayed timing gate 1.2 nsec, 89.1%.

&Sigma_cycles C^- : Total number of observed monochromatic peak counts on the Λ momentum spectrum: 73.6 ± 8.6

N^-_Kμ2 : cycle-by-cycle value of detected &mu^- number under the condition, T_delay > 1.2 nsec. 111561/111704/44124 for E549/E570-1/E570-2.

&epsilon^-_Λt : cycle-by-cycle value of physical acceptance of the (⁴He+K^-)_atomic->Λ+t reaction, to be finalized here.

In order to estimate physical acceptance &epsilon^-_Λt defined as,

N_detected = N_produced*&epsilon^-_Λt,

we perform a Monte-Carlo simulation of the cascade reaction, and conditions of the simulation are as tabulatted below. The physical acceptance includes

Geometrical acceptance by PA-PDC-PB-NT (proton),

Geometrical acceptance by PA-PDC (triton),

Geometrical accpetance by TC-VDC (&pi^-),

Branching ratio of Λ->p+π^- decay,

In-flight reaction (proton/π^-),

Analysis efficiency of proton selection by 1/&beta VS total energy at PB + NT (proton),

Efficiency of energy loss correction (p and π^-),

PA-PB timing gate, 45 nsec by E549/E570 electronics (proton),

and

Efficiency of the opening-angle selection, cos(Λt)<-0.99, for reconstructed 3-momentum of Λ smeared by multiple-scattering and TOF resolution for p and &pi^- on correspondig arms.

The condition of the Monte-Carlo for the acceptance calculation is tabulatted below:

Conditions of the Monte-Carlo.
software	GEANT3.21(partice tracking tuned for slow particles)
Reaction	(K^--⁴He)_atomic -> Y⁰+t
Branching ratio	50% (Y⁰ : Λ)/ 50% (Y⁰ : Σ⁰)
(K^--⁴He)_atomic life time	6.6*10^-17 sec (Γ=10 eV)
Generated number of (K^--⁴He)_atomic	2*10⁷
Target center (cm)	(x,y,z)=(-0.3,0.,1.3):E549 / (-0.5,0.5,1.2):E570
x/y generation point distribution	4.0 cm &sigma Gaussian centered at (x,y)=(-0.3,0.)
z generation point distribution	uniform
Multiple scattering	on(Moliere)
Energy loss straggling	on(Gauss/Landau/Vavilov are internally selected adequately)
In-flight nuclear reaction of p/t/π^-	on(GHEISHA, unique selection for in-flight reaction loss for d)
Coincidense time gate for PA-PB	45 nsec
Birk's coefficients	0.013/(MeV/cm), 9.6E-06/(MeV/cm)² for PA/PB/NT/TC
Time resolution of PA/PB	60/90 psec (~108.2 psec in total), fixed at MIP-measured value.
TOF resolution of &pi^- by PA-TC	250 psec.
Proton selection	Simulated with 1/&beta VS total Light output (with infinite energy resolution).
PDC/VDC hit	Only 1 particle is accepted per chamber. Multi track events (p+&pi^-,etc) are eliminated.
Inefficiency of energy loss correction	Considered to p and π^-. As the decay vertex, exact position is adopted. The direction vector at the PDC/VDC position is adopted as the direction vector to simulate properly the experimental effect.

Distributions of some quantities

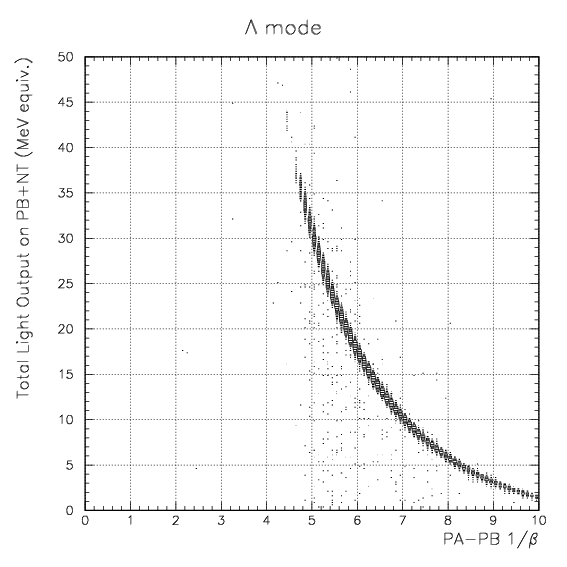
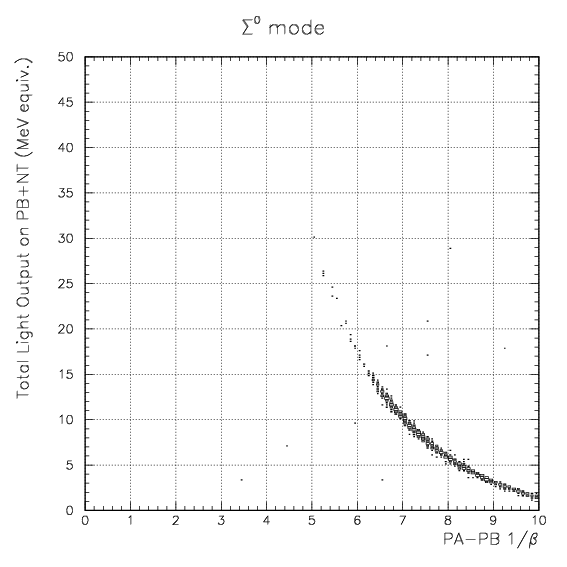
Since we are not very familiar to the 4NA process, we first try to simulate vrious quantities, to check the properties of the processes. In these processes, the range is so long compared to the tritons from ⁴_ΛHe -> tp decay, and hence a substatntial fraction is expected to reach the PB counter. Therefore, the triton selection introduced previously to select 508.4 MeV/c triton from ⁴_&LambdaHe -> tp decay is too narrow for these 4NA processes, and we do need to care additionally energetic part which is out of the present selection. The selection is performed by PAPB-measured 1/β VS energy deposit on PB, as was done for proton/deuteron.

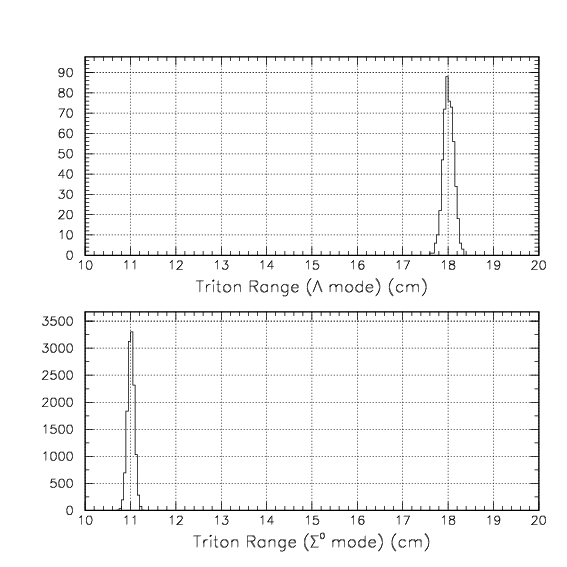
Simulated range distributions for both modes. They peak at ~18 (Λt) and ~11 (&Sigma⁰t) cm, respectively.

Simulated correlation of 1/β VS total energy deposit on PA, for Λt and &Sigma⁰t modes, respectively. The red events are those which reached PB.

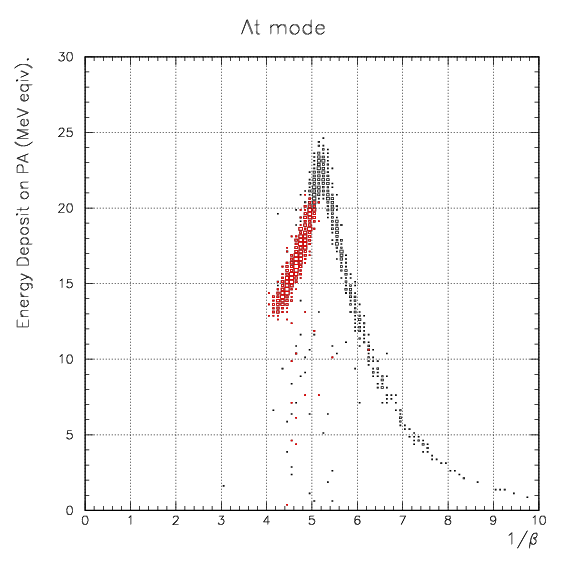
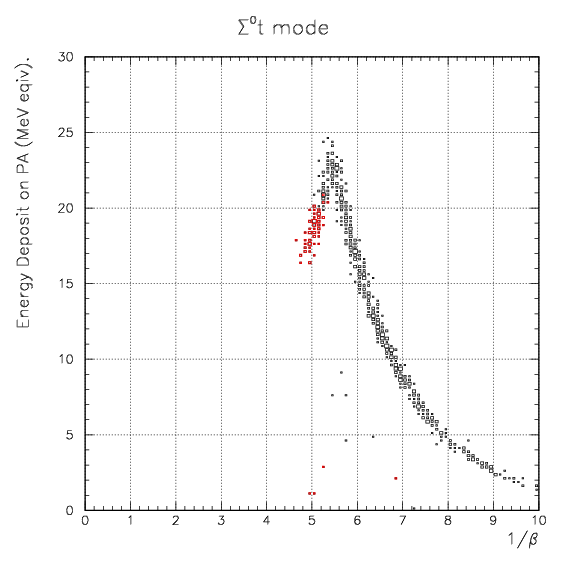
Simulated correlation of PAPB-measured 1/β VS total energy deposit on PB+NT, for Λt and &Sigma⁰t modes, respectively. The red events are those which reached PB.

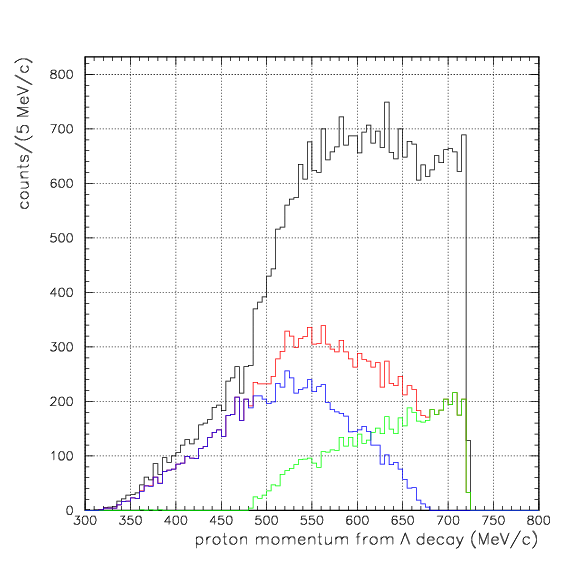
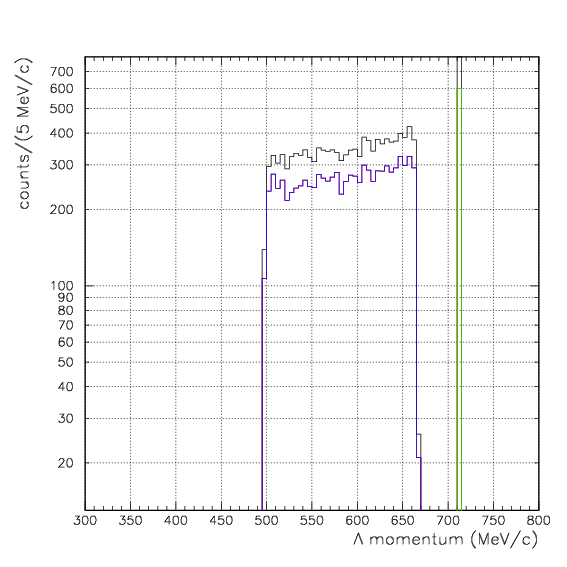
Simulated distribution of exact 3-momentum of proton and Λ under backward triton coincidence condition. The red is from stopped events on PA, and the green and the ble are from Λ and Σ⁰ modes, respectively.

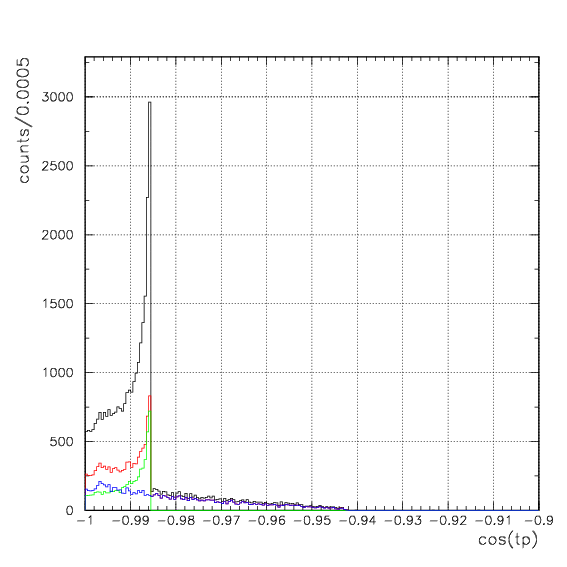
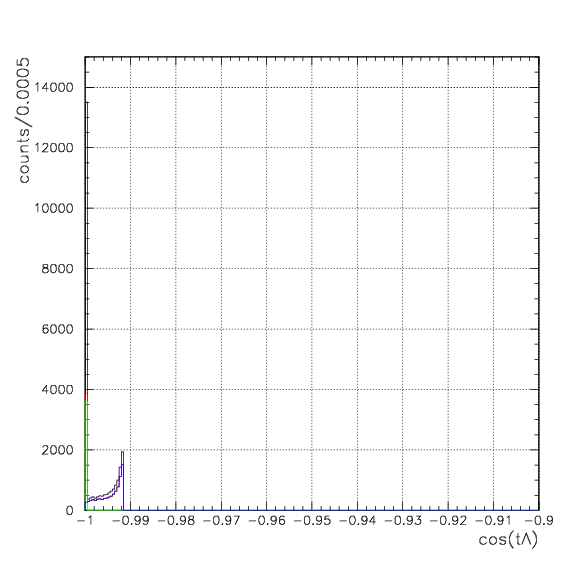
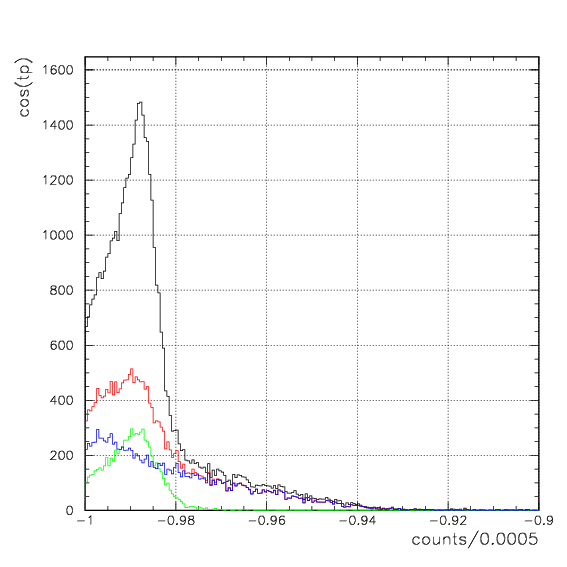
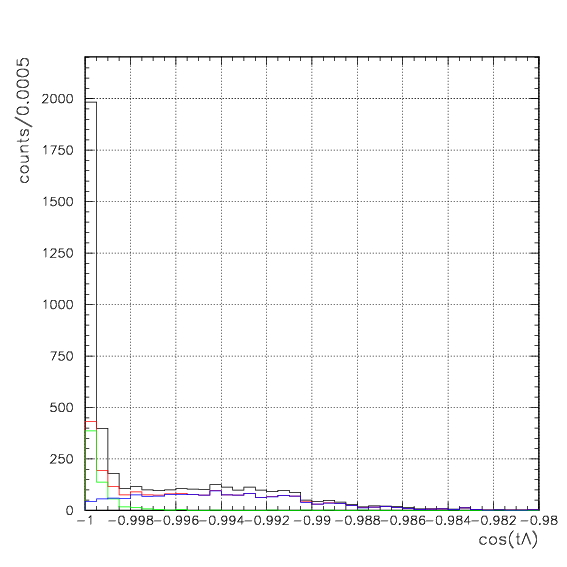
Simulated distribution of exact cos(tp) and cos(tΛ) under backward triton coincidence condition. The definition of colors are just as above.

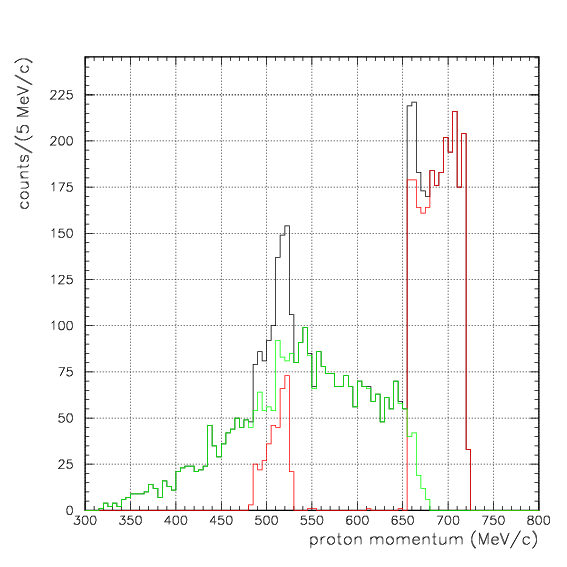
Simulated distribution of exact 3-momentum of proton for stopped triton events on PA. Note that cos(pt) < -0.990 has been imposed here. The red is by Λt events, while the green is by the Σ⁰t events. From the figure, it is easily explained that the observed valley at around 650 MeV/c in the backward triton coincidence proton spectrum is explained as the border between tΛ and t&Sigma⁰ processes.

Simulated cos(tp) distribution. Experimental effects are considered as tabulated. Here the red is from stopped triton events, and the green and the blue are from Λ and Σ⁰ branchs, respectively.

Simulated cos(tΛ) distribution. Experimental effects are exactly considered as tabulatted. The red is from stopped triton events, and the green, blue are by Λt and &Sigma⁰t events among the stopped set. The Λt events are all within cos(Λt)&le-0.998.

Simulated proton momentum spectrum under backward triton coincidence condition for stopped triton. Note that cos(tp) < -0.990 is imposed here. The red is from Λt, while the green is from &Sigma⁰t. This reproduce well the observed spectrum shape over 500 MeV/c.

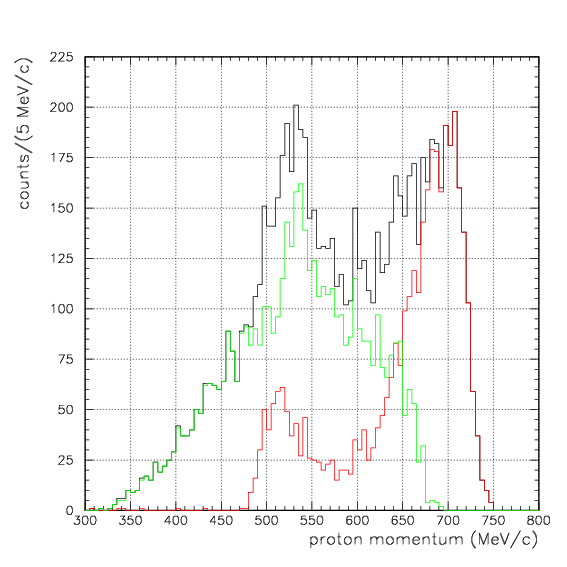
Simulated Λ momentum spectrum under backward triton coincidence condition for stopped triton events. Experimental effects are exactly considered as tabulatted. The red-filled is the spectrum obtained by cos(tΛ)≤-0.998, and its Gaussian fitting result is also shown.

Numerical Result and reaction branching ratio of the Λ t mode (preliminaly).

Here, we tabulate the numerical results of the acceptace calculation.

Generated and Accepted Event Numbers for tp mode (1)
Starting from the column 4, we add additional selection as indicated on the top row up to the 8th column. This table is a preliminary table for the subsequent one in which statistics are improved 100 times.
Cycle	reaction	generated event No.	PDC-PA-PB hit of p	.AND. no π^- hit on p-fired arm	PDC-PA hit of t	.AND.no pion hit on t-fired arm	time gate (45 nsec) + selection on analysis (p)
E549	Λt	1.91146E+06	88760	71950	40704	40693	28406
E549	&Sigma⁰t	1.91221E+06	88394	76454	22477	22468	18043
E570	Λt	1.89684E+06	88213	71616	40111	40100	27951
E570	&Sigma⁰t	1.89635E+06	88451	76221	19684	19680	15728

Generated and Accepted Event Numbers for tp Mode (2)
A continuation of the table above, but total statistics were improved by 100 times. All selections on the table above are applyed as the default. Starting from the column 2, we add additional selections as indicated on the top row up to the 6th. &epsilon_Λt is then just defined by the bold number in the 6th colmun divided by the number in the 1st colmun.
generated event No.	Successful energy loss correction (p)	π^- detection by TC-VDC	Successful energy loss correction(π^-)	1108.0<IM(pπ^-)<1124.0	cos(tΛ)<-0.99	&epsilon_Λt(%)
1.91304E+08	2.83782E+06	302561	287321	253125	249897	1.31E-01
1.91145E+08	1.78896E+06	251411	239335	212832	178445	9.34E-02
1.89604E+08	2.78384	292176	277537	243159	239929	1.27E-01
1.89734E+08	1.57905	219749	209425	185598	151111	7.96E-02

Adopting these evaluated acceptance values of &epsilon_Λt and observed peak counts of Λt events, we now obtain

Br((⁴He+K^-)_atomic -> Λ + t) = 3.2±0.5(syst)±0.4(stat)×10^-4.

However, we do need to improve the accuracy of the acceptance calculation or triton selection procedure, because triton selection efficiency is not exactly considered up to now. Here, we found that a large fraction of triton from t+Λ process reach to PB, and hence triton selection procedure must be improved in the data analysis, because the present procedure developed in 2010 May 6th report invented to search for ⁴_ΛHe-> tp is not to lose stopped triton on PA, so that the realistic efficiency of the passing-through triton is unknown, but the accurate derivation is not feasible and unneeded considering the present accuracy of other quantities. In order to check whether significant systematic deviation is found or not, we will try to accumulate as much triton as possible to apply the ε_Λt derived above, and try to check the consistency between the other result obtained only by PA-PB-identified triton, to which the corresponding acceptance can be calculated more accurately. Here we present the second efficiency:

&epsilon¹_Λt: The efficiency when only tritons detected by PA-PB 1/β VS ΔE on PB are accumulated. This efficiency is to be applied to the future detection number obtained for "triton" events on PA-PB.

The calculated efficiency, &epsilon¹_Λt are presented below, as the continuation of the tables above.

triton on PB / &epsilon¹_Λt(%)
114663/5.99E-02
9281/4.86E-03
97525/5.14E-02
4569/2.41E-03

In the next report, we will try to identify the triton on PA-PB and apply the ε¹_Λt presented above, to check whether the result is consistent to the overall result obtained by the all triton events and ε_Λt.

A comparison of the measured and expected peak center and width

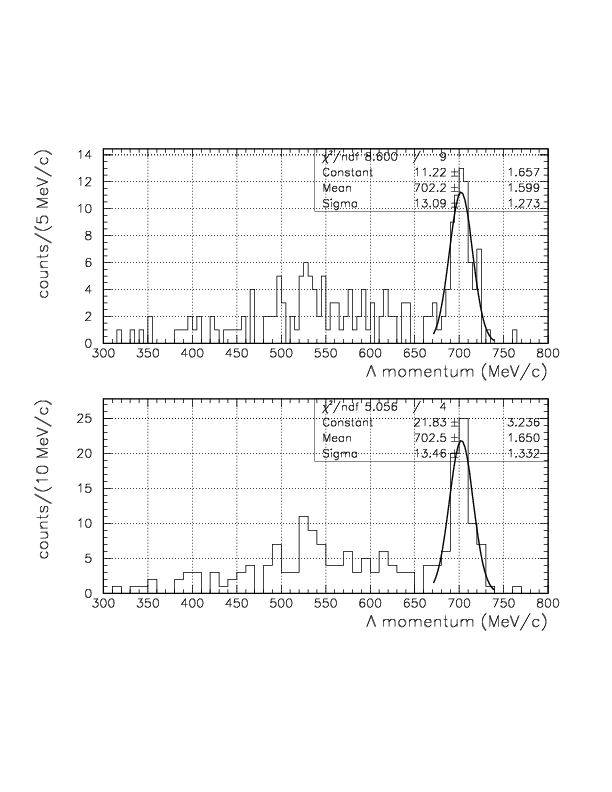
Performing naively a Gaussian fitting of the measured momentum peak of Λ, we obtain the peak area, center and the standard deviation of 73.6 ± 8.6, 702.5 ± 1.5 and 13.5 ± 1.5 MeV/c, respectively. The peak area is consistent to the value by a naive integratuion. By some reason, the peak center is systematicly shifted by 9.2 ± 1.5 MeV/c toward the lower side, and the resolution value is consistent to the calculated value of ~12 MeV/c.

Today's Progress 11. May 2010

2010 May 11th report - The evaluation of per-stopped-K- formation rate of (4He-K-)atomic -> &Lambda + t four-nucleon absorption process (1)

Normalization of the process and acceptance study

Conditions of the Monte-Carlo.

Distributions of some quantities

Simulated range distributions for both modes. They peak at ~18 (Λt) and ~11 (&Sigma0t) cm, respectively.

Simulated correlation of 1/β VS total energy deposit on PA, for Λt and &Sigma0t modes, respectively. The red events are those which reached PB.

Simulated correlation of PAPB-measured 1/β VS total energy deposit on PB+NT, for Λt and &Sigma0t modes, respectively. The red events are those which reached PB.

Simulated distribution of exact 3-momentum of proton and Λ under backward triton coincidence condition. The red is from stopped events on PA, and the green and the ble are from Λ and Σ0 modes, respectively.

Simulated distribution of exact cos(tp) and cos(tΛ) under backward triton coincidence condition. The definition of colors are just as above.

Simulated cos(tp) distribution. Experimental effects are considered as tabulated. Here the red is from stopped triton events, and the green and the blue are from Λ and Σ0 branchs, respectively.

Simulated cos(tΛ) distribution. Experimental effects are exactly considered as tabulatted. The red is from stopped triton events, and the green, blue are by Λt and &Sigma0t events among the stopped set. The Λt events are all within cos(Λt)&le-0.998.

Simulated proton momentum spectrum under backward triton coincidence condition for stopped triton. Note that cos(tp) < -0.990 is imposed here. The red is from Λt, while the green is from &Sigma0t. This reproduce well the observed spectrum shape over 500 MeV/c.